On October 29, 1998, at 2 pm, an announcement was read by a Christie’s representative to the audience gathered in the display room at 20 Rockefeller Plaza, New York: “We are pleased to inform our clients that the Federal Court of New York last night denied a motion form the Greek Orthodox Patriarchate of Jerusalem, to enjoin the sale of Archimede’s Palimpsest.” Bidding started soon after, and the precious booklet, a small in-octavo collection of 174 parchment sheets bound in a worn leather cover, amply showing its more than 1,000 years of age, was sold for 2,2 million dollars to a mysterious buyer, which the press currently identifies with the Amazon founder and CEO Jeff Bezos. For Bezos, the richest man in the world, such a momentous purchase amounted to 0,001 per cent of his own net worth. Comparing such figure to my own salary, it would have been like me going out to buy a croissant at the local bakery.
Archimede’s Palimpsest contains, among other documents, the only known copy in original Greek of his treaty “Okoumenon”, or On Floating Bodies. The book has a story that would easily make for a Hollywood action movie with major actors. It was copied around 950 AD in Constantinople from a previous manuscript dated around 530, written by Isidorus of Miletus. The copy was salvaged from the sack of Constantinople in 1204 (the Crusaders, in their faith-instilled fury, burned all the books written in Greek as “blasphemous”), by christian monks, and remained in possession of the Greek Orthodox Church at least until 1920. In the meantime, the original parchment sheets had been disbanded, and some pious monk had carefully scraped them and reused for writing a book of prayers, which was then transported together with other stuff from Jerusalem back to Constantinople (now Istanbul) and kept in the Methiochon (the central library of the Church).
Fortunately, the original text was still barely visible under the overwritten liturgy, and when the most famous Archimedean scholar of his times, the Danish Johan Heiberg, had the chance of viewing it in 1906, he immediately decided it was an Archimede’s original. The manuscript disappeared after the Greco-Turkish war of 1918-22, and reappeared in Paris around 1930, in the hands of the businessman Marie-Louis Sirieix, who pretended to have purchased it from some monk, however without proof. In the following years, his heirs tried to sell privately the book, now substantially ruined and degraded, until they were forced to hand it to Christie’s for a public auction. It was only at that point that the Orthodox Church attempted to reclaim rights on the book, affirming it had been stolen, but it was eventually sold as described above. The new owner, however, has let the precious book free for study, reproduction and analysis, and several scholars could finally reconstruct the treatise about floating bodies from the closest possible version to Archimede’s original, which was actually written in form of letters the great scientist addressed to his friends to recount his ingenious findings.
Archimede’s principle and the buoyant force are taught in all schools at different levels, and everybody uses a practical knowledge of the floating principle when going to take a swim in the sea. The legend tells that Archimedes was charged by the Geron, the tyrant king of Siracusa, at that time the richest Greek colony in Sicily, of deciding whether a gold crown he had crafted from a local goldsmith was really made of gold. The king suspected the artisan could have stolen some of the gold, and replaced it with some more vile metal, like silver or copper. According to the legend, Archimedes found the solution while bathing, and observing that the immersion of his own body in water displaced an amount of fluid, after which he jumped out of the bath and ran naked in the streets of Siracusa, yelling Eureka! (“I found it!”). He then devised a balance to weight an equal mass of gold against the crown, and proved that the crown volume displaced a larger amount of water, thereby showing it was made of metals less dense than gold. The legend is probably not true, because such a measurement would have required a very high accuracy, barely available at that time. Let us assume that the crown weighted 1 kg, that is about 50 cm3 of volume for a gold density of 19,8 g/cm3, and was put in a container of about 20 cm diameter. The fully submerged crown would have raised the water surface by barely 0,16 cm. Even if as much as half of the gold was replaced by copper, to a density of 14,4 g/cm3 and a volume of about 70 cm3, this fake crown would raise the water level by 0,22 cm: could Archimedes – yet ingenious, however living in the 5th century BC – spell out a difference of 60 microns?
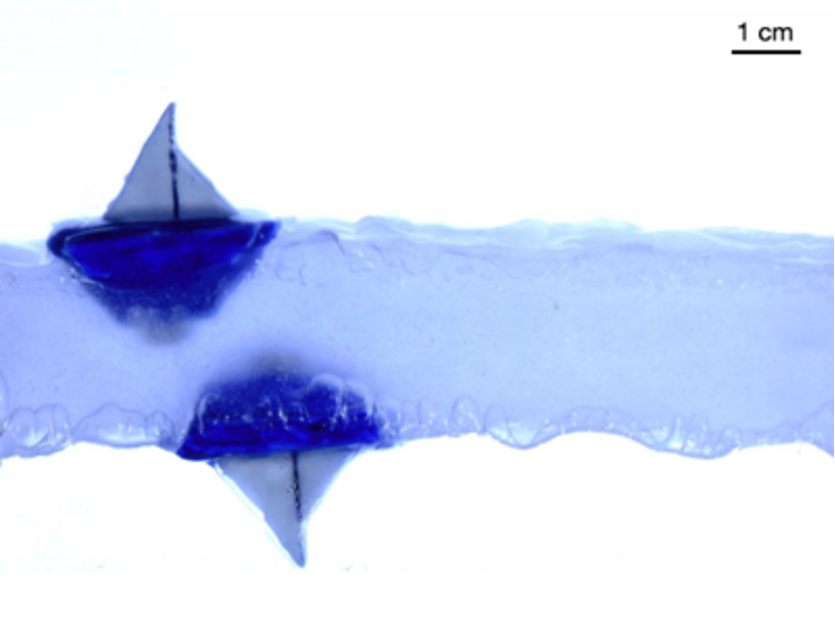
This Sunday’s Nature paper by Apffel at al. reports a curious effect of “floating under a levitating fluid”. As you can read yourself, by arranging a series of experiments in which they make layers of fluids to levitate over empty space, they observe a variety of interesting phenomena, among which a stable buoyancy at the lower free surface. The fluid used is a high-density, high-viscosity one, such as glycerol or silicone, in a glass container subject to a vertical vibration at frequencies of the order of 100 Hz. According to Archimede’s 2,300-years old findings, an object of density lower than the fluid, deposited on the surface, would sink to the maximum depth at which the weight of fluid displaced equals its weight, and thus float above the surface. However, for a levitating layer of fluid, a second free surface exists below. Counter-intuitively, the same object delicately placed at the lower surface, experiences equally the cancellation of its weight by the buoyant force, and remains floating on the lower surface “upside down” (see Figure 2 in the paper and movies in the Supplementary Material). This second equilibrium position is metastable: the object could fall down, or slide to the upper surface, if slightly moved from the equilibrium position. However, the authors show that the dynamical effect provided by the vibrations provides an extra stability to this singular effect, that can be filmed for quite a long time.
This beautifully demonstrated upside-down floating is not the only strange effect about Archimede’s principle. As early as the 1960s, it was noticed that the apparent protein density in solution varied depending on the nature of the solvent. The discrepancies prompted researchers to ask what effect the fluid complexity could have, on the buoyant force felt by an object. Should the weight of displaced fluid in Archimedes’s principle be that of the bare solvent, or that of the suspension? It turns out that when the fluid is a complex colloidal suspension or a highly structured solvent, the amount of displaced fluid can be significantly altered by the density perturbations that a particle induces in its surroundings.[1]
To see how, imagine adding a single large object (a protein) to a bunch of smaller molecules suspended in water (e.g. Percoll or sucrose). In equilibrium, the smaller particles experience a buoyant force that balances their own weight. But when the test object is introduced into the same volume, the distribution of those particles is no longer uniform, and changes in a way that depends on their mutual interactions with the object: the interactions might include van der Waals, coulombic, hydrogen bonds, hard-sphere and so on. Interactions produce a particle concentration profile described by a radial distribution function, g(r), which quantifies the local deviation from uniform density. Therefore, the actual weight of fluid displaced in a given volume is proportional to some integral of the distribution function, and not just to the difference in densities.
Moreover, Archimede’s principle does not include the surface tension 𝛴. We all have seen sometimes insects (“water striders”) with long legs L walking on water, thanks to the additional lift provided by the curved surface making a contact angle with the legs. The forces acting on the insect are the gravity, G=Mg; buoyancy, B= gV; and surface tension S=Lcos𝜃. Surface tension can be much larger than the buoyant force for very elongated objects, with volume V=R2L. In The Physics of Living Systems (see Chap. 11, p.481-2), I defined a non-dimensional quantity S/B as being proportional to the ratio R/L, to establish that the long legs of water striders (L>>R) can indeed provide enough lift S to overcome both B and G. Surface tension introduces a shape dependence in Archimede’s principle: namely, if you hammer a sphere of iron into a very thin sheet, surface tension will be able to make it float, even if its volume and weight are the same as that of a sphere that would, instead, immediately sink.
([1]) R. Piazza et al., Soft Matter (2012) 8, 7112
